If you’ve ever been taught about how Earth orbits around the Sun, you might well think our planet travels along an oval-shaped path that brings it much closer to the Sun at some times of the year than at others. You’d have a good reason to think that, too: It’s how most textbooks show things.
Indeed, many people assume Earth is closer to the Sun in summer than in winter. As it happens, this is true during summer in the southern hemisphere, but it can’t also be true for summer in the northern hemisphere.
In the southern hemisphere, Earth is 5 million kilometers closer to the Sun in summer than in winter, but it’s the reverse in the northern hemisphere. The average Earth-Sun distance is 150 million kilometers, and the main reason for the seasons is Earth is tilted, so each pole is sometimes pointing more toward the Sun and sometimes more away from it.
So Earth’s orbit only has a relatively tiny deviation from perfect circularity. But why is it so often shown as practically an egg shape? And how can we visualize the real situation?
Consider the bike wheel
In order to try to understand myself how circular the orbit of the Earth was and other planets, I decided to compare the shape of Earth’s orbit to an ordinary 26-inch bike wheel by scaling down the real dimensions to fit — and consulting my local bike shop about what the deviations would mean for a real wheel. I was very surprised at the result.
The orbit was far closer to a perfect circle than I had previously thought. If the orbit were a 26-inch (660.4mm) bicycle wheel, the deviation from a perfect circle would be less than 0.1mm. That’s comparable to a thin coat of paint — essentially indistinguishable from a perfect circle to the naked eye.

I looked at the other planets, too. The orbits of Venus and Neptune are even closer to perfect circles, with the orbit of Venus deviating only 14μm (a μm or micrometer is a millionth of a meter) and Neptune 31μm.
The planets with the least circular orbits are Mars and Mercury. If the orbit of Mars were a 26-inch bike wheel, it would be out by just less than 3mm — hardly noticeable if you were riding a bike with a wheel out of true by this amount.
Mercury has the least circular of the orbits, with a deviation of 14mm, although this is still only 2 percent.
If you have a bike, chances are its wheels are not even as circular as Mars’s orbit. If you’ve had a decent collision with a curb or rock, your front wheel might even be less circular than the orbit of Mercury.
A tiny deviation of Earth’s orbit
Mathematically minded readers might have a question after reading the above: if Earth is on average 150 million kilometers from the Sun, and this distance varies by 5 million kilometers over the course of a year, shouldn’t the deviation in its orbit be a little over 3 percent?
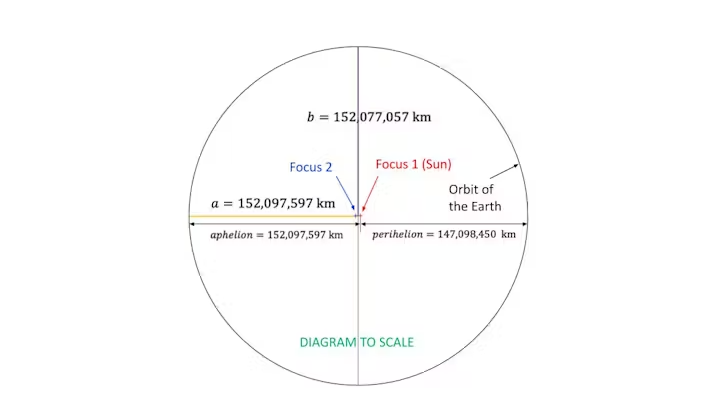
The answer to this question is that the Sun is not at the center of the ellipse, but offset to one side as a point called the focus. If, during formation, a planet traveled at just the right speed to counteract gravity, it would travel in a circle.
However, in the real universe, planets rarely go at just the right speed for a circle. Sometimes, they travel a bit faster and sometimes slower, which can only be achieved with an elliptical orbit.
Coming full circle
Thousands of years ago, the ancient Greeks believed all celestial objects orbited around the Earth, traveling in perfect circles.
This idea held sway for about 1,500 years, until Polish astronomer Nicolaus Copernicus (1473-1543) realized the planets (including Earth) actually orbited around the Sun.
Copernicus thought the orbits were circular. Later, German astronomer and mathematician Johannes Kepler (1571-1630) realized he was wrong and devised the three laws of planetary motion.
The first law states that planets’ orbits are elliptical rather than circular. The third law links the size of a planet’s orbit to the amount of time it takes in a way that’s a bit too complicated for us to discuss here.
The second law is that if you draw line from the Sun to any given planet, the line will sweep out equal areas in equal amounts of time as the planet moves. Think of pizza — a narrow wedge of a large pizza can have the same area as a wide wedge of a small pizza. This happens because planets move faster when they are closer to the Sun.
The main reason why orbits are drawn as ellipses in textbooks is to demonstrate Kepler’s second law. If the orbit of the Earth was drawn as shown in the correctly scaled diagram, it would be impossible to see any difference in the wedges.
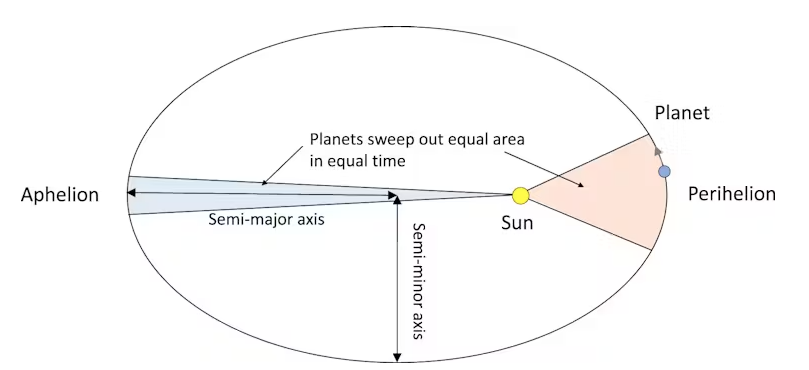
However, this can give the impression the orbit of the Earth is far more elliptical than it actually is. Such diagrams are not actually wrong — they are an exaggeration, a kind of mathematical caricature that emphasizes an important feature.
Although the ancient Greeks were wrong about the Earth being at the center of the solar system, they were not far wrong about the orbits of the planets. So, if you’ll excuse the pun, we have come full circle.
Stephen Hughes, Honorary Senior Lecturer, School of Mathematics and Physics & UQ College, The University of Queensland
This article is republished from The Conversation under a Creative Commons license. Read the original article.
Follow us on X, Facebook, or Pinterest

